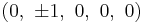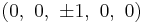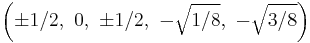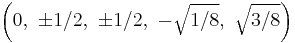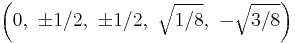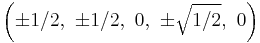Stericated 5-simplex

Orthogonal projections in A₅ and A₄ Coxeter planes
5-simplex	Stericated 5-simplex
Steritruncated 5-simplex	Stericantellated 5-simplex
Stericantitruncated 5-simplex	Steriruncitruncated 5-simplex
Steriruncicantitruncated 5-simplex (Omnitruncated 5-simplex)

In five-dimensional geometry, a stericated 5-simplex is a convex uniform 5-polytope with 4th order truncations (sterication) of the regular 5-simplex.

There are 6 unique sterications of the 5-simplex, including permutations of truncations, cantellations, and runcinations. The simplest stericated 5-simplex is also called an expanded 5-simplex, with the first and last nodes ringed, for being constructable by an expansion operation applied to the regular 5-simplex. The highest form, the steriruncicantitruncated 5-simplex is more simply called a omnitruncated 5-simplex with all of the nodes ringed.

Stericated 5-simplex

Stericated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,4{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62	12 {3,3,3} 30 {}×{3,3} 20 {3}×{3}
Cells	180	60 {3,3} 120 {}×{3}
Faces	210	120 {3} 90 {4}
Edges	120
Vertices	30
Vertex figure	Tetrahedral antiprism
Coxeter group	A₅ [[3,3,3,3]], order 1440
Properties	convex, isogonal, isotoxal

A stericated 5-simplex can be constructed by an expansion operation applied to the regular 5-simplex, and thus is also sometimes called an expanded 5-simplex. It has 30 vertices, 120 edges, 210 faces (120 triangles and 90 squares), 180 cells (60 tetrahedra and 120 triangular prisms) and 62 hypercells (12 pentachora, 30 tretrahedral prisms and 20 3-3 duoprisms).

Its 30 vertices represent the root vectors of the simple Lie group A₅. It is the also the vertex figure of the 5-simplex honeycomb.

Alternate names

Expanded 5-simplex
Stericated hexateron
Small cellated dodecateron (Acronym: scad) (Jonathan Bowers)^[1]

Cross-sections

The maximal cross-section of the stericated hexateron with a 4-dimensional hyperplane is a runcinated pentachoron. This cross-section divides the stericated hexateron into two pentachoral hypercupolas consisting of 6 pentachora, 15 tetrahedral prisms and 10 3-3 duoprisms each.

Coordinates

The vertices of the stericated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,1,1,1,1,2). This represents the positive orthant facet of the stericated hexacross.

The Cartesian coordinates in 5-space for the normalized vertices of an origin-centered stericated hexateron are:

$\left(\pm1,\ 0,\ 0,\ 0,\ 0\right)$

$\left(0,\ \pm1,\ 0,\ 0,\ 0\right)$

$\left(0,\ 0,\ \pm1,\ 0,\ 0\right)$

$\left(\pm1/2,\ 0,\ \pm1/2,\ -\sqrt{1/8},\ -\sqrt{3/8}\right)$

$\left(\pm1/2,\ 0,\ \pm1/2,\ \sqrt{1/8},\ \sqrt{3/8}\right)$

$\left( 0,\ \pm1/2,\ \pm1/2,\ -\sqrt{1/8},\ \sqrt{3/8}\right)$

$\left( 0,\ \pm1/2,\ \pm1/2,\ \sqrt{1/8},\ -\sqrt{3/8}\right)$

$\left(\pm1/2,\ \pm1/2,\ 0,\ \pm\sqrt{1/2},\ 0\right)$

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[[5]]=[10]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[[3]]=[6]

orthogonal projection with [6] symmetry

Steritruncated 5-simplex

Steritruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,2,3{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62	6 t_0,1{3,3,3} 15 {}xt_0,1{3,3} 20 {3}x{6} 15 {}x{3,3} 6 t_0,2{3,3,3}
Cells	330
Faces	570
Edges	420
Vertices	120
Vertex figure
Coxeter group	A₅ [3,3,3,3], order 720
Properties	convex, isogonal

Alternate names

Steritruncated hexateron
Celliprismated hexateron (Acronym: cappix) (Jonathan Bowers)^[2]

Coordinates

The coordinates can be made in 6-space, as 180 permutations of:

(0,1,1,1,2,3)

This construction exists as one of 64 orthant facets of the steritruncated hexacross.

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[5]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[3]

Stericantellated 5-simplex

Stericantellated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,2,4{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62	12 t02{3,3,3} 30 t02{3,3}x{} 20 {3}x{3}
Cells	180	60 t02{3,3} 240 {}x{3} 90 {}x{}x{} 30 t1{3,3}
Faces	900	360 {3} 540 {4}
Edges	720
Vertices	180
Vertex figure
Coxeter group	A₅ [[3,3,3,3]], order 1440
Properties	convex, isogonal

Alternate names

Stericantellated hexateron
Celliprismatotruncated dodecateron (Acronym: captid) (Jonathan Bowers)^[3]

Coordinates

The coordinates can be made in 6-space, as permutations of:

(0,1,1,2,2,3)

This construction exists as one of 64 orthant facets of the stericantellated 6-orthoplex.

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[[5]]=[10]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[[3]]=[6]

Stericantitruncated 5-simplex

Stericantitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,1,2,4{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62
Cells	480
Faces	1140
Edges	1080
Vertices	360
Vertex figure
Coxeter group	A₅ [3,3,3,3], order 720
Properties	convex, isogonal

Alternate names

Stericantitruncated hexateron
Celligreatorhombated hexateron (Acronym: cograx) (Jonathan Bowers)^[4]

Coordinates

The coordinates can be made in 6-space, as 360 permutations of:

(0,1,1,2,3,4)

This construction exists as one of 64 orthant facets of the stericantitruncated 6-orthoplex.

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[5]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[3]

Steriruncitruncated 5-simplex

Steriruncitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,1,3,4{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62	12 t_0,1,3{3,3,3} 30 {}xt_0,1{3,3} 20 {6}x{6}
Cells	450
Faces	1110
Edges	1080
Vertices	360
Vertex figure
Coxeter group	A₅ [[3,3,3,3]], order 1440
Properties	convex, isogonal

Alternate names

Steriruncitruncated hexateron
Celliprismatotruncated dodecateron (Acronym: captid) (Jonathan Bowers)^[5]

Coordinates

The coordinates can be made in 6-space, as 360 permutations of:

(0,1,2,2,3,4)

This construction exists as one of 64 orthant facets of the steriruncitruncated 6-orthoplex.

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[[5]]=[10]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[[3]]=[6]

Omnitruncated 5-simplex

Omnitruncated 5-simplex
Type	Uniform 5-polytope
Schläfli symbol	t_0,1,2,3,4{3,3,3,3}
Coxeter-Dynkin diagram
4-faces	62	12 t_0,1,2,3{3,3,3} 30 {}×t_0,1,2{3,3} 20 {6}×{6}
Cells	540	360 t{3,4} 90 {4,3} 90 {}x{6}
Faces	1560	480 {6} 1080 {4}
Edges	1800
Vertices	720
Vertex figure	irr. {3,3,3}
Coxeter group	A₅ [[3,3,3,3]], order 1440
Properties	convex, isogonal, zonotope

The omnitruncated 5-simplex has 720 vertices, 1800 edges, 1560 faces (480 hexagons and 1080 squares), 540 cells (360 truncated octahedrons, 90 cubes, and 90 hexagonal prisms), and 62 hypercells (12 omnitruncated 5-cells, 30 truncated octahedral prisms, and 20 6-6 duoprisms).

Alternate names

Steriruncicantitruncated 5-simplex (Full description of omnitruncation for 5-polytopes by Johnson)
Omnitruncated hexateron
Great cellated dodecateron (Acronym: gocad) (Jonathan Bowers)^[6]

Permutohedron

The omnitruncated 5-simplex is the permutohedron of order 6. It is also a zonotope, the Minkowski sum of six line segments parallel to the six lines through the origin and the six vertices of the 5-simplex.

Orthogonal projection, vertices labeled as a permutohedron.

Related honeycomb

Like all uniform omnitruncated n-simplices, the omnitruncated 5-simplex can tessellate space by itself, in this case 5-dimensional space with 3 facets around each ridge. It has Coxeter-Dynkin diagram of .

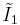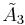Coxeter group	${\tilde{I}}_{1}$	${\tilde{A}}_{2}$	${\tilde{A}}_{3}$	${\tilde{A}}_{4}$	${\tilde{A}}_{5}$
Coxeter-Dynkin
Picture
Name	Apeirogon	Hextille	Omnitruncated 3-simplex honeycomb	Omnitruncated 4-simplex honeycomb	Omnitruncated 5-simplex honeycomb
Facets

Coordinates

The vertices of the truncated 5-simplex can be most simply constructed on a hyperplane in 6-space as permutations of (0,1,2,3,4,5). These coordinates come from the positive orthant facet of the steriruncicantitruncated 6-orthoplex, t_0,1,2,3,4{3⁴,4}, .

Images

orthographic projections
A_k Coxeter plane	A₅	A₄
Graph
Dihedral symmetry	[6]	[[5]]=[10]
A_k Coxeter plane	A₃	A₂
Graph
Dihedral symmetry	[4]	[[3]]=[6]

Stereographic projection

Related uniform polytopes

These polytopes are a part of 19 uniform polytera based on the [3,3,3,3] Coxeter group, all shown here in A₅ Coxeter plane orthographic projections. (Vertices are colored by projection overlap order, red, orange, yellow, green, cyan, blue, purple having progressively more vertices)

t₀	t₁	t₂	t_0,1	t_0,2	t_1,2	t_0,3
t_1,3	t_0,4	t_0,1,2	t_0,1,3	t_0,2,3	t_1,2,3	t_0,1,4
t_0,2,4	t_0,1,2,3	t_0,1,2,4	t_0,1,3,4	t_0,1,2,3,4

Notes

^ Klitizing, (x3o3o3o3x - scad)
^ Klitizing, (x3x3o3o3x - cappix)
^ Klitizing, (x3o3x3o3x - card)
^ Klitizing, (x3x3x3o3x - cograx)
^ Klitizing, (x3x3o3x3x - captid)
^ Klitizing, (x3x3x3x3x - gocad)

References

H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
  - (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
  - (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  - (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
Richard Klitzing, 5D, uniform polytopes (polytera) x3o3o3o3x - scad, x3x3o3o3x - cappix, x3o3x3o3x - card, x3x3x3o3x - cograx, x3x3o3x3x - captid, x3x3x3x3x - gocad

External links


Family	A_n	BC_n	D_n	E₆ / E₇ / E₈ / F₄ / G₂	H_n
Regular polygon	Triangle	Square		Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	5-cell	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	1₂₂ • 2₂₁
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	1₃₂ • 2₃₁ • 3₂₁
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	1₄₂ • 2₄₁ • 4₂₁
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
n-polytopes	n-simplex	n-orthoplex • n-cube	n-demicube	1_k2 • 2_k1 • k₂₁	pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes

Stericated 5-simplex

Contents